The length of the second fundamental form, a tangency principle and applications

MATHEMATICAL SCIENCES

The length of the second fundamental form, a tangency principle and applications

Francisco X. Fontenele^I; Sérgio L. Silva^II

^IUniversidade Federal Fluminense, Instituto de Matemática, Departamento de Geometria 24020-140 Niterói, RJ, Brasil

^IIUniversidade Estadual do Rio de Janeiro-UERJ, Departamento de Estruturas Matemáticas-IME 20550-013 RJ, Brasil

^{Correspondence}

ABSTRACT

In this paper we prove a tangency principle (see Fontenele and Silva 2001) related with the length of the second fundamental form, for hypersurfaces of an arbitrary ambient space. As geometric applications, we make radius estimates of the balls that lie in some component of the complementary of a complete hypersurface into Euclidean space, generalizing and improving analogous radius estimates for embedded compact hypersurfaces obtained by Blaschke, Koutroufiotis and the authors. The basic tool established here is that some operator is elliptic at points where the second fundamental form is positive definite.

Key words: hypersurfaces, tangency principle, second fundamental form, balls, radius estimates.

RESUMO

Neste trabalho nós provamos um princÍpio de tangência (veja Fontenele and Silva 2001) para hipersuperfícies de um espaço ambiente arbitrário e relacionado com o comprimento da segunda forma fundamental. Como aplicações geométricas, nós fazemos estimativas dos raios das bolas contidas em uma determinada componente conexa do complemento de uma hipersuperfÍcie completa do espaço Euclidiano, generalizando e melhorando estimativas de raios análogas obtidas por Blaschke, Koutroufiotis e os autores. O fato básico estabelecido aqui é que um determinado operador é elÍptico nos pontos onde a segunda forma fundamental é positiva definida.

Palavras-chave: hipersuperfícies, princípio de tangência, segunda forma fundamental, bolas, estimativas de raios.

1 INTRODUCTION

In (Fontenele and Silva 2001), the same authors proved that if an n-dimensional embedded compact hypersurface Mⁿ into (n+1)-dimensional Euclidean space satisfies |H_k| > , for some k-mean curvature H_k, 1 < k < n, and some positive constant l, then the greatest ball that fits inside Mⁿ has radius less than l unless Mⁿ is a sphere of radius l, generalizing results of (Blaschke 1956) and (Koutroufiotis 1973) for surfaces. Our basic tool for the proof of the above result was a tangency principle stated in (Fontenele and Silva 2001) as Theorem 1.1. This tangency principle turned out to be very useful to obtain other geometric applications. In this work we obtain a tangency principle (see Fontenele and Silva 2001) related with the length of the second fundamental form and improve (see Remark 3.1) the radius estimate for the greatest ball that fits inside a suitable component of the complementary of an n-dimensional complete hypersurface into (n+1)-dimensional Euclidean space. In order to state our results we need the following.

As in (Fontenele and Silva 2001), given a hypersurface Mⁿ of a complete Riemannian manifold Nⁿ⁺¹ with metric á , ñ and exponential mapping exp: TN ® N, we parametrize a neighborhood of Mⁿ containing p and contained in a normal ball of Nⁿ⁺¹ putting

where x varies in a neighborhood W of zero in T_pM (the tangent space to Mⁿ at p), h_o is a fixed unitary vector normal to Mⁿ at p and m is an unique real function defined in W with m(0) = 0. Let h: W ® M be a local orientation of Mⁿ with h(0) = h_o . Denote by A_h(x) the second fundamental form of Mⁿ in the direction h(x) and by s the vector valued second fundamental form of Mⁿ. The length of the second fundamental form at x is given by

If l₁ (x) < l₂ (x) < ¼ <l _n (x) are the principal curvatures of Mⁿ at x Î W, we have that

Given hypersurfaces and of Nⁿ⁺¹ with T_pM₁ = T_pM₂ (tangent at p), parametrize and as in (1) obtaining correspondent functions m¹ and m². As in (Fontenele and Silva 2001), we say that remains above in a neighborhood of p with respect to h_o if m¹ (x) > m ² (x) in a neighborhood of zero.

Following the ideas in (Fontenele and Silva 2001), we obtain the following tangency principle:

THEOREM 1.1. Consider hypersurfaces

and

of Nⁿ⁺¹ tangent at p and h_o a unitary vector normal to

at p. Denote by |s₁|²(x) and |s₂|²(x) the length of the second fundamental form of respectively

and

at x Î W. Assume that

remains above

in a neighborhood of p with respect to h_o , |s₁|²(x)< |s₂|²(x) in a neighborhood of zero and that the principal curvatures of M₂ at zero are all positive. Under these conditions,

and

must coincide in a neighborhood of p.

For hypersurfaces with boundaries and as a consequence of proof of Theorem 1.1, we obtain the following tangency principle:

THEOREM 1.2. Let

and

be hypersurfaces of Nⁿ⁺¹ with boundaries ¶M₁ and ¶M₂ respectively. Suppose that

and

as well as ¶M₁ and ¶M₂ are tangent at p Î ¶M₁ǶM₂ and let h_o be normal to

at p. Denote by |s₁|²(x) and |s₂|²(x) the length of the second fundamental form of respectively

and

at x Î W. Assume that

remains above

in a neighborhood of p with respect to h_o , |s₁|²(x)< |s₂|²(x) in a neighborhood of zero and that the principal curvatures of M₂ at zero are all positive. Under these conditions,

and

must coincide in a neighborhood of p.

When the ambient is the (n+1)-dimensional Euclidean space and the hypersurfaces and have the same constant length of the second fundamental form, Theorems 1.1 and 1.2 are the analogous of the well known maximum principles for hypersurfaces with the same constant k-mean curvature in the Euclidean space.

For enunciate our geometric applications let us fix some notation. Given an oriented hypersurface Mⁿ of the (n+1) - dimensional Euclidean space 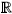ⁿ+1, the k-mean curvature H_k(p) of Mⁿ at p is given by

where l₁< l₂< ¼ < l _n are the principal curvatures of Mⁿ at p. In particular, H₁(p) is denoted by h(p) and called the mean curvature of M at p and H₂(p) is denoted by R(p) and called the scalar curvature of M at p.

The Ricci curvature of Mⁿ at a point p in the direction of an unitary vector u is given by

where w₁ = u, w₂,... ,w_n is an orthonormal basis of T_pM and K(u,w_i) is the sectional curvature of the plane generated by u and w_i.

DEFINITION 1.3. If U is an open subset of the Euclidean space 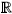ⁿ⁺¹, we define

r_u := sup{ r >0, such that contains a closed ball in 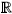ⁿ⁺¹ of radius r},

where denotes the closure of U in 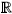ⁿ⁺¹.

CONDITION I. Mⁿ is a complete connected euclidean hypersurface that splits 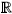ⁿ⁺¹ into two disjoint regions of which Mⁿ is the common boundary.

THEOREM 1.4. Suppose that Mⁿ satisfies Condition I. Assume further that |s|² > and |h| > over Mⁿ, where l is a positive constant. Under these conditions, if we denote by U the component of

ⁿ⁺¹\M that contains the normals for which h is positive, then r_U< l. Moreover, if U È M contains a closed ball of radius l then Mⁿ coincides with a sphere in

ⁿ⁺¹ of radius l.

COROLLARY 1.5. Suppose that Mⁿ satisfies Condition I. Assume further that |s|²>

and R >

over Mⁿ, where l is a positive constant. Then, the mean curvature function h is positive for some suitable orientation of Mⁿ and, denoting by U the component of

ⁿ⁺¹\M that contains the normals for which h is positive, we have r_U< l. Moreover, if in the closure of U there exists a closed ball of radius l, then Mⁿ must be a sphere in

ⁿ⁺¹ of radius l.

COROLLARY 1.6. Suppose that Mⁿ satisfies Condition I and that |H_k| >

on Mⁿ for some k, 1 < k < n, and some positive constant l > 0. For k > 2, assume further that there exists at least one point in Mⁿ where the second fundamental form is definite. Then there exists a component U of

ⁿ⁺¹\M such that r_U< l. Moreover, if UÈM contains a closed ball of radius l, then Mⁿ coincides with a sphere in

ⁿ⁺¹ of radius l.

THEOREM 1.7. Suppose that Mⁿ satisfies Condition I and is oriented. Assume that h > 0 and that |s|²>

over Mⁿ, where l is a positive constant. Assume further that n > 3 and Ric_M> —

. Then, if we denote by U the component of

ⁿ⁺¹\M that contains the normals, we have r_U<l. Moreover, if UÈMⁿ contains a closed ball of radius l then either n must be even or Mⁿ coincides with a sphere in

ⁿ⁺¹ of radius l.

COROLLARY 1.8. Suppose that Mⁿ satisfies Condition I and that |s|²>

over Mⁿ, for some positive constant l. Consider also that Ric_M > —. Then, the mean curvature function h is positive for some suitable orientation of Mⁿ and denoting by U the component of 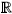ⁿ⁺¹\M that contains the normals for which h is positive, we have r_U<l. Furthermore, if in the closure of U there exists a closed ball of radius l, then Mⁿ must be a sphere in

ⁿ⁺¹ of radius l.

In the following result, Mⁿ is a connected and complete manifold isometrically immersed in 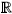ⁿ⁺¹.

COROLLARY 1.9. Assume that K_M> 0 and |s|²>

over Mⁿ, for some positive constant l. If Mⁿ is not compact, assume further that there exists at least one point in Mⁿ where all sectional curvatures are positive. Then there exists a component U of

ⁿ⁺¹\M such that r_U<l. Moreover, if UÈM contains a closed ball of radius l then Mⁿ coincides with a sphere in

ⁿ⁺¹ of radius l.

2 SKETCH OF PROOF OF THEOREM 1.1

Fix an orthonormal basis e₁,e₂,... ,e_n in T_pM₁ = T_pM₂ and introduce coordinates, for x in T_pM₁, setting x = x_i e_i. As in , parametrize M₁ and M₂ in a neighborhood of p by respectively j¹ and j² , obtaining respectively functions m¹ and m². Let h^l : W® M_l, l = 1,2, be a local orientation of M_l with h^l(0) = h_o and denote by the second fundamental form of M_l in the direction h^l(x). Denote by (x) the vector (x) and by A^l(x) the matrix of in the basis (x), 1 < i < n. In (Fontenele and Silva 2001), it is proved the existence of a matrix valued function à defined in , where is a connected open set of 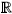ⁿ⁺¹ containing the origin, such that

where ((x),(x),m^l(x),x), 1 < i < j < n, is a point of 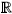^d, d = +2n+1. We write an arbitrary point of 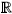^d as (r_ij,r_i,z,x), 1 < i < j < n, and x = (x₁,¼,x_n). Define a function F: ® 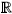by

Now using the derivatives of Ã, with respect to the r_kl's, given in (Fontenele and Silva 2001), we obtain that

where A^t(0) = Ã( (1-t) (0)+t (0) , 0 , 0 , 0 ). Using that remains above in a neighborhood of p with respect to h_o , |s₁|²(x) < |s₂|²(x) in a neighborhood of zero and that the principal curvatures of M₂ at zero are all positive, one can prove that F is elliptic in ((1-t) (0)+t (0) , 0 , 0 , 0 ) for t Î [0,1], and, restricting W if necessary, conclude that F is elliptic with respect to the functions (1-t)m²+ tm¹, t Î [0,1] (see Fontenele and Silva 2001).

Recalling that |s_l|²(x) = trace [A^l(x)]², it follows from (2), (3) and our assumptions that

Now the conclusion of the theorem is obtained from the following maximum principle (Alexandrov 1962):

MAXIMUM PRINCIPLE. Let f, g: U ® 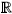be C²-functions defined in an open set U of 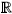ⁿ and let F: G Ì 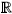^d® 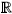be a function of class C¹ . Suppose that F is elliptic with respect to the functions (1-t)f+tg, t Î [0,1]. Assume also that

and that f < g on U. Then, f < g on U unless f and g coincide in a neighborhood of any point x_o Î U such that f (x_o ) = g(x_o).

Now we will prove Theorem 1.7 for give an idea of how one can use Theorem 1.1 to obtain geometric applications.

3 PROOF OF THEOREM 1.7

PROOF OF THEOREM 1.7. Consider in

ⁿ⁺¹ an arbitrary closed ball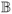 _r[p_o], centered at p_o and radius r, contained in U È M. Move 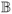_r[p_o] until its boundary 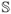_r [p_o] touches Mⁿ the first time. Let p be a tangency point between Mⁿ and 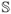_r [p_o]. Denote by l₁< l₂< ¼< l _n the principal curvatures of Mⁿ at p. It is well known that l_i < 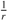for all i. Since l₁ +l₂+¼+l_n> 0 by assumption, we have

where means that l_i has been omitted on the sum. Therefore, for a negative l_i, we deduce that

where e_i stands for an unitary eigenvector with eigenvalue l_i. The above inequality, gives -< l _i < 0 for a negative l_i. We consider two possibilities:

P.1. There exists at p at least one negative l_i. Denoting by t the number of l_i^'s that are negative and using our assumption on the length of the second fundamental form, we get

Thus, l> r. In case r = l, we have that l₁ = l₂ = ¼ = l_t = - and l_t+1 = ¼ = l_n = . Since h > 0, we obtain that n > 2 t. On the other hand, we have

which implies that n < 2t. Hence, n = 2 t and n is even. Notice also that Mⁿ is minimal at p and the Ricci curvature at p is constant and equal to -.

P.2. At p, all l_i's are nonnegative. In this case,

Thus l> r. If r = l, it follows easily that l₁ = l₂ = ¼ = l_n = . Using Theorem 1.1 and noting that is the constant value of the length of the second fundamental form of a sphere having radius r, we obtain that Mⁿ and 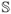_r [p_o] coincide in a neighborhood of p. By an argument of connectness, we conclude that Mⁿ is equal to 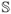_r [p_o].

REMARK 3.1. We point out that Corollary 1.6 extends to complete hypersurfaces Theorem 1.3 in (Fontenele and Silva 2001) and that its hypothesis are stronger than those in Theorem 1.4. In fact, for k = 1, this follows from the well known inequality |s|²> n h² and, for k > 1, this follows from Lemma 1 in (Montiel and Ros 1991). Furthermore, the estimate for r_U in Theorem 1.4 improves the one given by Corollary 1.6. For if inf|h| = > 0 and inf|s|² = with |h|> , the upper bound given for r_U in Theorem 1.4 is l_o and the one given by Corollary 1.6 is H_o. That l_o< H_o follows from the well known inequality |s|²> n h². 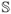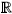For example, in the cilinder C = ¹ × in 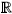³, oriented by the normals pointing to the component U of 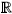³ \C containing the origin,where 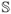¹ is the unitary circle, the mean curvature and length of the second fundamental form are given respectively by and 1. The estimate given by Theorem 1.4 is r_U < , while the estimate given by Corollary 1.6 is r_U< 2.

Manuscript received on September 5, 2003;

Accepted for publication on November 21, 2003;

Presented by Manfredo do Carmo

The first author dedicates this work to his parents José (in memoriam) and Herondina

• ALEXANDROV AD. 1962. Uniqueness theorems for surfaces in the large I-V, Amer Math Soc Transl, Ser. 2, 21: 341-416.
• BLASCHKE W. 1956. Kreis und Kugel. 2. Auflage, Berlin.
• FONTENELE F AND SILVA SL. 2001. A tangency principle and applications, Illinois J Math, 45: 213-228.
• KOUTROUFIOTIS D. 1973. Elementary geometric applications of a maximum principle for nonlinear elliptic operators, Arch Math, 24: 97-99.
• MONTIEL S AND ROS A. 1991. Compact hypersurfaces: the Alexandrov theorem for higher order mean curvatures, Differential Geometry (Lawson B and Tenenblat K, eds.), 52, Pitman Monographs, Essex, Longman: 1-13.

Publication Dates

• Publication in this collection
  17 Feb 2004
• Date of issue
  Mar 2004

History

• Accepted
  21 Nov 2003
• Received
  05 July 2003