Abstracts
In the context of the Lagrangian and Hamiltonian mechanics, a generalized theory of coordinate transformations is analyzed. On the basis of such theory, a misconception concerning the superiority of the Hamiltonian formalism with respect to the Lagrangian one is criticized. The consequent discussion introduces the relationship between the classical Hamilton action and the covariance properties of equations of motion, at the level of undergraduate teaching courses in theoretical mechanics.
transformation coordinates; Lagrangian and Hamiltonian functions; classical action; covariance
Uma teoria generalizada de transformações de coordenadas é analisada no contexto da mecânica em termos da lagrangiana e hamiltoniana. Com base nessa teoria, critica-se um equívoco que ressalta a superioridade do formalismo hamiltoniano em relação ao formalismo lagrangiano. A discussão decorrente introduz a relação entre a ação clássica de Hamilton e as propriedades de covariância das equações do movimento no nível adequado para o ensino de graduação em mecânica teórica.
transformações de coordenadas; funções hamiltoniana e lagrangiana; ação clássica; covariância
ARTIGOS GERAIS
Transformation properties of the Lagrange function
Propriedades de transformação da função de Lagrange
Carlo FerrarioI,1 1 E-mail: ferrario@fe.infn.it. ; Arianna PasseriniII
IDepartment of Physics and INFN Section, University of Ferrara, Ferrara, Italy
IIDepartment of Engineering, University of Ferrara, Ferrara, Italy
ABSTRACT
In the context of the Lagrangian and Hamiltonian mechanics, a generalized theory of coordinate transformations is analyzed. On the basis of such theory, a misconception concerning the superiority of the Hamiltonian formalism with respect to the Lagrangian one is criticized. The consequent discussion introduces the relationship between the classical Hamilton action and the covariance properties of equations of motion, at the level of undergraduate teaching courses in theoretical mechanics.
Keywords: transformation coordinates, Lagrangian and Hamiltonian functions, classical action, covariance.
RESUMO
Uma teoria generalizada de transformações de coordenadas é analisada no contexto da mecânica em termos da lagrangiana e hamiltoniana. Com base nessa teoria, critica-se um equívoco que ressalta a superioridade do formalismo hamiltoniano em relação ao formalismo lagrangiano. A discussão decorrente introduz a relação entre a ação clássica de Hamilton e as propriedades de covariância das equações do movimento no nível adequado para o ensino de graduação em mecânica teórica.
Palavras-chave: transformações de coordenadas, funções hamiltoniana e lagrangiana, ação clássica, covariância.
1. Introduction
The contents of recent advanced textbooks in classical mechanics [1-5] seem to denote that several theoretical physicists are now engaged in making more intelligible the applications of a formalism which, developed by mathematicians, was becoming maybe too abstract. We are referring to the intrinsic description of the mechanics given by the differential geometry, which brings into focus in a sharp way the concepts of invariance and covariance of physical laws. Without resorting to the differential geometry methods, it is possible to point out some of those concepts so enriching the traditional teaching approach.
With such perspective, the main purpose of the present paper is to make clear the equivalence between Lagrangian and Hamiltonian formalisms, by discussing the transformation properties and the invariance conditions of the classical action ∫
dt. To this aim we will identify the most wide class of transformations which maintain the Euler-Lagrange structure of the equations of motion and we will revisit the theory of generating functions in the Hamiltonian framework. The results exposed throughout the present paper are addressed both to stimulate the teaching of the classical mechanics in an undergraduate course, as well as to give a sound starting point for the transformation rules in quantum mechanics for any advanced course in theoretical physics.From the traditional teaching point of view (see for instance famous textbooks as [6,7]), Hamiltonian Mechanics looks more general than the Lagrangian one, since its covariance transformations constitute a wider class with respect to point transformations. Such a didactic setting, although justified by important developments such as Hamilton-Jacobi theory, induces a severe misconception which may go beyond the classical mechanics. Actually, Lagrangian and Hamiltonian approaches to quantization are distinct and independent, and no conclusive preference can be given to canonical quantization rather than to Feynman's path integral. It is easy to imagine that classical non equivalence would lead to non equivalent quantum theories.
In sec. 2, we start to point out the complete equivalence, by introducing non point Lagrangian transformations of the equations of motion, leading to a dynamics which can still be Lagrangian. We emphasize that the second order character of any Lagrangian dynamics is an essential feature to be preserved in a transformation. We end by noticing that the inclusion of non point transformations excludes, in general, the possibility to have a scalar field as Lagrange function.
In sec. 3, we show that non point transformations preserving the structure of Lagrange equation are nothing else than canonoid transformations (and, in particular, canonical). A necessary and sufficient condition for a map to be canonoid is discussed and specialized to canonical maps, inferring also a fundamental relation between the Lagrangians.
In sec. 4, with the aid of elementary examples, we recover the invariance of the Lagrangian by focusing the relevant conditions on the canonoid transformations. In addition we propose a weak condition of invariance of the Lagrangian, and discuss the consequences of such occurrence in terms of the Hamiltonian action. Finally we analyze a simple example of quantum canonical transformation.
2. Theory of the transformations and scalar invariance of the Lagrangian
One could say that all the equivalence between the Lagrangian and the Hamiltonian formalisms lies on the following features:2 2 Here, we will assume that Lagrange's equations of motion are second order differential equations, which can be written in normal form.
1. the representative spaces are equivalent from the topological point of view; more, the velocity space and the phase space are diffeomorphic manifolds [5];
2. a dual transformation between the spaces links the functions of Lagrange (
) and Hamilton (H), containing all the information on the dynamics of the system, as well as the equations of motion [8];
3. in both cases the equations of motion follow from a variational principle; in fact, they are Euler-Lagrange equations of two (different) variational problems.
Though a theory of the transformations in Lagrangian mechanics could in principle be characterized by the request that after a change of variables the equations of motion preserve the structure of Lagrange equations, in literature the natural maps are point transformations.
The latter map the configuration space onto itself; as a consequence, the velocities which are tangent to the trajectories at a given point, become vectors of the tangent space in the transformed point. In such a way, an essential property of the equations is preserved: they are still of the second order and can be put in normal form. Therefore, the dynamics is deterministic, once the initial conditions are given.
Further, as a direct consequence of the Hamilton principle (see Ref. [7]), the Lagrange equations are covariant (that is, they preserve the form of Euler-Lagrange equations) if one performs a point transformation.
Moreover, the Lagrangian associated with the new equations is still the old one. This means that the Lagrangian is an intrinsic object, univocally defined once the geometry of the configuration space and the mechanical properties of the system are given. So, one says that the Lagrange function behaves like a scalar under a point transformation; namely, the Lagrangian changes its functional dependence on the coordinates but its numerical value in a given point remains the same. We will speak of scalar invariance to express this outcome. In coordinates
On the other hand, as we will discuss in sec. 3, a phase space transformation maintains the Hamiltonian form of the equations of motion provided it is canonoid [5] with respect to a particular Hamiltonian. If, in addition, it preserves the Poisson brackets, it is canonical. Moreover, it is well known that the point transformations in phase space belong to a proper subset of the canonical transformations.3 3 The most elementary proof is given by the exchange transformation Q = p, P = -q (see Ref. [7]), which is non point but canonical. This depends on the fact that canonical transformations are identified as those preserving the form of all Hamiltonian dynamics, which, as Euler-Lagrange equations exhibit more general covariance properties. On the other hand, when one performs such a change of variables, it may occur that the new generalized coordinate Q, depending also on the old momentum p, is unsuitable for the local description of the configuration space.
For this reason, the complete correspondence between the two formalisms seems not full, at least concerning the theory of covariance transformations.
In the present paper, the equivalence between the formalisms is recovered provided one asks that in the velocity space the transformed dynamics is still Lagrangian, although not necessarily with respect to the same Lagrangian. As we will see, this leads to include in the theory those transformations known as canonoid.
Let us take a Lagrangian 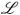 (q,
(q, 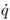 , t). Then, we write the Lagrange equation as two first order differential equations in normal form
, t). Then, we write the Lagrange equation as two first order differential equations in normal form
with
Starting from Eq. (2), with a slight abuse of notation, 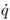 denotes the coordinate of the tangent space at q and not the time derivative of q. In place of the notation u =
denotes the coordinate of the tangent space at q and not the time derivative of q. In place of the notation u = 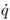 , from which Eq. (2) should be written as
, from which Eq. (2) should be written as
we prefer to keep a more familiar notation and recall that the present dynamical description is carried out on a 2n dimensional space and not on the configuration space.
Now, let us consider the most general transformation (q, 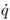 ) → (Q,
) → (Q,  ), say
), say
and assume that it can be inverted. Next, differentiating (4) with respect to time, we have
We demand that once we have rewritten the system (2), the new equations maintain the same normal form, in which one of the variables is just the velocity, while the second equation furnishes the acceleration. Such a condition is known in literature as Second Order Differential Equation condition [9], and if we impose it in Eq. (5), we get
This condition allows a certain freedom, without constraining us to the point transformation. The problem is possibly another: how to derive the equations in the new variables from a variational principle? The so-called inverse problem in the calculus of variations [10] establishes the condition of existence of a Lagrange function once a second order equation is given.
Let us face a simple example in which our problem possesses a solution.
Example 1. Let us consider the one-dimensional motion of a particle with unitary mass, under the influence of a constant force. The Lagrangian of the system being 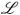 =
= 
Let us perform the following non point transformation
and make use of Eqs. (5) and (6). Consequently, we obtain the new second order equation
Equations (10) can be immediately derived from the Lagrangian  .
.
Three points about this example are important to be noticed:
(a) the transformation (9) satisfies the second order condition for the system (8), but not necessarily for any Lagrange equation; as a counterexample the free particle equation
= 0 is sufficient;
(b) besides the different functional form, the two Lagrangians have different values in the same point, as one can verify by direct substitution of Eq. (9) in
; no kind of invariance arises.
(c) there is no reason to think a priori that
is a scalar with respect to a non point transformation; as a matter of fact, if we substitute in
the new coordinates according to Eq. (9), we obtain a Lagrangian non equivalent to
: it gives rise to equations completely unrelated with dynamics (10).
The example can induce to think that there is no general method to solve this kind of problem, and that the procedure used in finding the new Lagrangian is by trial and error. On the contrary, the generalized transformations we are proposing are connected with known properties of the Hamiltonian formalism, as we will see in the next section. Moreover, the condition of solvability of the inverse problem in the Hamiltonian framework are simpler (see the Poisson bracket theorem in Ref. [5, p. 222-224]).
We will show that such transformations are always related to maps that are if non canonical at least canonoid.
3. The Hamiltonian framework
Hence, let us assume that a map of the kind (4), enjoying condition (7) for a dynamics described by 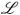 (q,
(q, 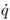 , t), transforms the equations of motion, leading to a dynamics described by
, t), transforms the equations of motion, leading to a dynamics described by  (Q,
(Q,  , t). Example 1 shows that in general
, t). Example 1 shows that in general
where (Q,  ) should be obviously evaluated by means of Eq. (4).
) should be obviously evaluated by means of Eq. (4).
The Hamiltonian characterization of the transformations we are studying can be obtained by composing two Legendre mappings and a transformation defined in the phase space, as summarized in the following scheme, in which we are assuming that all the maps are invertible:
If we construct non point transformations in this way, then relations (4) preserve the structure of Lagrangian dynamics for the dynamics generated by 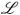 (q,
(q, 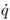 , t), but not necessarily the Lagrangian.
, t), but not necessarily the Lagrangian.
Thus, we consider the function (q, p) → (Q, P), corresponding in the phase space to the transformation (4), as a mapping acting first of all on the equations of motion. Then, the existence of a Hamiltonian K(Q, P, t) does not imply that the transformation is canonical: it is sufficient that the equations derived from the particular
are transformed in equations which keep the canonical form.
In literature, such transformations are known as canonoid with respect to H [11]. And one could say that the canonical transformations are those maps which are canonoid with respect to all Hamiltonians.
For instance, the map (9) of Example 1 becomes
in the phase space. The transformed differential equations can be derived by the Hamiltonian K = 2P2 - Q. Since the fundamental Poisson bracket [Q, P]q, p is not equal to 1, this map is canonoid with respect to H =  p2 - q, but non canonical.
p2 - q, but non canonical.
The above scheme provides a synthetic representation of the relationship between the descriptions we are considering: given a transformation connecting the two dynamics of the Lagrangian functions 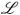 (q,
(q, 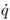 , t) and
, t) and  (Q,
(Q,  , t), one finds two Hamiltonian functions with their equations of motion connected by a canonoid map. And viceversa given H(q, p, t) and K(Q, P, t) one can always come back to a couple of Lagrange equations, which are obviously second order differential equations.
, t), one finds two Hamiltonian functions with their equations of motion connected by a canonoid map. And viceversa given H(q, p, t) and K(Q, P, t) one can always come back to a couple of Lagrange equations, which are obviously second order differential equations.
At this point, we have to remind that asking covariance for Hamilton equations means to keep fixed the statement of the variational principle, while changing the variables
Consequently (see for instance Ref. [7]), from the arbitrariness of the variations, one can be sure to get the same Hamiltonian dynamics if a function F defined in the phase space exists such that
This condition is sufficient but not necessary for the covariance of the Hamilton equations: as a matter of fact, the integrands in Eq. (14) can differ by any function  which, for the given particular Hamiltonian H, identically satisfies the Euler-Lagrangian equations in phase space
which, for the given particular Hamiltonian H, identically satisfies the Euler-Lagrangian equations in phase space
In principle  may have an expression as
may have an expression as
where the ci are arbitrary multiplicative constants and the symbol 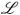 e means equivalent Lagrangian [10] to
e means equivalent Lagrangian [10] to 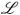 , in the sense that any solution of the equations of motion obtained from
, in the sense that any solution of the equations of motion obtained from 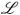 verifies also the ones obtained by
verifies also the ones obtained by 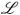 e.4
4
To be rigorous, we should write
p
-
H in place of
, but the equivalence between the two formalisms is by now sufficiently clear.
We hence underline that condition (15) is necessary and sufficient for canonicity, whereas it is generalized by
e.4
4
To be rigorous, we should write
p
-
H in place of
, but the equivalence between the two formalisms is by now sufficiently clear.
We hence underline that condition (15) is necessary and sufficient for canonicity, whereas it is generalized by
in case of canonoid transformations. The essential difference is based on the fact that canonical transformations leave covariant any Hamiltonian dynamics.
To better understand the role of  , let us return to Example 1. By using the dynamics equations of the example, in particular
, let us return to Example 1. By using the dynamics equations of the example, in particular  = 1, it is easy to verify that both the following expressions are correct
= 1, it is easy to verify that both the following expressions are correct
Consequently, both the choices (0,0,1) and (1,0,-1) are admissible for (c1,c2,c3).
Finally, we can derive a necessary and sufficient condition of existence for a canonoid transformation, as equation for  . In fact, by differentiating (19) with respect to q and successively with respect to p, and taking into account that
. In fact, by differentiating (19) with respect to q and successively with respect to p, and taking into account that
we obtain
In this way we have proved the following
Proposition 1: a transformation (q, p) → (Q, P) is canonoid with respect to H if and only if a function  exists satisfying
exists satisfying
This condition is exactly Eq. (3.2) in Ref. [12]: there,  is called generating function and the result is obtained from a geometric viewpoint, while we derived it by varying the action.
is called generating function and the result is obtained from a geometric viewpoint, while we derived it by varying the action.
Finally, in order to introduce the subject of the next section, let us consider those canonical transformations whose generating function is a constant of motion (for a given dynamics): we remark that since of Eq. (15) the Lagrangian behaves as a scalar. In particular, we return to point transformations: in the Hamiltonian formalism, a point transformation is a canonical transformation with the property
because of the scalar nature of 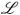 . Moreover, Eq. (28) does not simply mean that F is a constant of motion, because it must hold true for every Hamiltonian dynamics. Then F is just a constant.
. Moreover, Eq. (28) does not simply mean that F is a constant of motion, because it must hold true for every Hamiltonian dynamics. Then F is just a constant.
In our generalization (19), we have an analogous invariance of 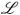 if
if  = 0. We analyze this invariance in the next section.
= 0. We analyze this invariance in the next section.
To conclude this section we briefly discuss the invariance properties of the Hamiltonian. We know from the textbooks that the Hamiltonian function is a scalar for time-independent canonical transformations. On the other hand, for canonoid transformations this property does not hold true and we prove in AppendixAppendix, the following
Proposition 2: A time-independent canonoid but non canonical transformation never leaves invariant its Hamiltonian.
4. Invariance of the action
Herein, we considered the most general class of covariance both for Hamiltonian and Lagrangian mechanics and proved that neither the Hamiltonian nor the Lagrangian functions are, in general, scalar fields. It is just the case to underline that it is hard to call "function" something which is not even a scalar field
How can our analysis naturally lead to some simple result concerning a theory of transformations in quantum mechanics? In facing such topics a student could naturally be lead to ask whether the classical theory of transformations, as we exposed it, still holds. Are change of variables and quantization of physical systems commutating procedures?
The general answer is "no", and a large amount of papers, see Refs. [13-16] just to give some examples are continuously produced on this complicated subject, as well as on the equivalence between Feynman's and canonical quantization [17-19].
Just to explain, and roughly speaking, canonical transformations need to possess a unitary counterpart allowing to transform Heisenberg's equations together with Hamilton's ones; but this occurs only provided the correspondence between the commutators is full.
In the present paper, we limit ourselves to point out some simple features of the Lagrangian framework, connected with the concept of invariance, which allow the use in quantum mechanics of some particular transformations of coordinates.
In the previous section, when dealing with classical mechanics, we asked the invariance of the condition
which identifies the real solutions among all possible curves in the configuration space .
Then, if one has the aim to preserve Feynman's path integral through a change of coordinate, it is natural, as a first step, to concentrate the attention on those transformations leaving unchanged the image of the functional
evaluated over sets of arbitrary curves.
This is clearly a stronger condition and, actually, only a few transformations can be adapted to the quantum framework.
By looking at the results collected in the previous section, one can perform a first attempt to get the invariance of Eq. (30) through a change of coordinates, by resorting to the scalar invariance of the Lagrangian. More, one can even consider a simple generalization of invariance of the Lagrangian, which reflects a possibly generalized invariance of A. To this end, we recall that if we multiply by a number the Lagrange function and add a gauge term to it (i.e. the time derivative of a function independent of the 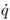 ), then the equations of motion are invariant. Therefore, the condition
), then the equations of motion are invariant. Therefore, the condition  = 0, ensuring the invariance of
= 0, ensuring the invariance of 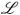 after a canonoid transformation, can be weakened by requiring in Eq. (18) that c2 = 0 and F = f(q, t). So one gets
after a canonoid transformation, can be weakened by requiring in Eq. (18) that c2 = 0 and F = f(q, t). So one gets
In case the canonoid transformation gives raise to Eq. (31), we will say that 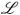 is a weakly invariant scalar field under the given transformation.
is a weakly invariant scalar field under the given transformation.
The weak changes induced in the action A by the ones we have just allowed in definition (31) are associated also with the invariance of the quantum description of the system. In particular, on one hand, we allow multiplication of the Lagrangian by a number since it can be absorbed in the functional A by scaling the time unit. On the other hand, whenever the configuration space is connected a gauge term can always be added to the Lagrangian without affecting the path integral [10]. The latter can be deduced by noticing that if A is increased with a constant independent of the particular curve (which is the case), then the quantum wave function is multiplied by a constant phase factor.
By considering only canonical transformations, from Eqs. (15) and (31) it follows, as a particular outcome, that the time derivative of the generating functions fulfils
which is the necessary and sufficient condition for a canonical transformation to leave a given 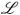 weakly invariant.
weakly invariant.
The strong invariance of 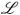 , as a particular case of Eq. (31), seems to restitute to the Lagrangian function the feature of intrinsic object we underlined in the context of point transformation. The same could be argued for the action which we remember to be a complete integral of Hamilton-Jacobi Eq. [7]. This could be the synthesis of our results from the classical viewpoint.
, as a particular case of Eq. (31), seems to restitute to the Lagrangian function the feature of intrinsic object we underlined in the context of point transformation. The same could be argued for the action which we remember to be a complete integral of Hamilton-Jacobi Eq. [7]. This could be the synthesis of our results from the classical viewpoint.
Let us show that the occurrence of Eq. (31) is not so rare: we exhibit a simple example of (strong) scalar invariance for 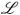 under a genuinely canonoid transformation.
under a genuinely canonoid transformation.
Example 2. Let us consider the transformation
which is canonoid with respect to the Hamiltonian H =  of the free particle. In fact, the transformed equations of motion are
of the free particle. In fact, the transformed equations of motion are
which are Hamilton equations of motion for the function
We emphasize that the transformation is not canonical because of [Q, P] = 2ln p, and nevertheless, there is no difference between the old and the new Lagrangian as scalar functions
On the other hand, an example of canonical transformation leaving invariant a given Lagrangian (as scalar field) is the following.
Example 3. Let us study the dynamics described by the Lagrangian
which trivially leads to a Hamiltonian
It is easy to verify that the canonical transformation
is generated by the function F = qeQ = qp, and that F is constant during the motion generated by H. Thus, since Eq. (15) holds true, we have that both the Lagrangian and the Hamiltonian are scalar. In addition, we underline that this canonical transformation, with the corresponding one induced in the velocity space, changes the functional form of the Hamiltonian and of the Lagrangian. In particular, the new equations of motion describe the free particle.
In the quantum framework, a class of allowed canonical transformations is that of canonical point transformations, as noticed by Jordan [20] from the very beginning. And, if we consider the Lagrangian point of view, the invariance of Eq. (30) under point transformations is an evident consequence of the scalar invariance of 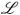 . This subject is fully developed, for instance, in Ref. [21].
. This subject is fully developed, for instance, in Ref. [21].
We conclude by presenting a known [22], less trivial, but simple example of canonical transformation possessing a unitary counterpart. It is an homogeneous linear canonical transformation, belonging to the symplectic group Sp(2), locally isomorphic to the (2 + 1)-dimensional Lorentz group. The weak scalar invariance of the Lagrangian as defined in Eq. (31) is verified for this transformation.
Example 4. From the point of view of quantum mechanics, it is possible to verify, by means of the PSD (phase space distribution) function W(q, p, t), that the transformation we are going to study describes the spread of the gaussian wave-packet of the free particle, through the deformation of the error box. Since the area (of the error box) is invariant, a characteristic feature of canonical maps comes out to be meaningful also in the quantum context.
Let us consider a free particle with unitary mass 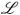 =
=  . If we perform the canonical transformation
. If we perform the canonical transformation
generated by the function
where F2 = F + PQ (see Ref. [7]), since F1(q, Q) does not exist in the present case, the new Hamiltonian is
The inverse Legendre map gives both  =
=  =
= 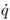 2, which clearly enjoys weakly scalar invariance, and the non point transformation
2, which clearly enjoys weakly scalar invariance, and the non point transformation
which verifies (6). The weak invariance of the Lagrangian could be proved also by directly calculating (as is always possible for canonical transformations)
and this expression enjoys property (32).
5. Conclusions
It has been recalled that point transformations and canonical transformations are characterized by geometrical properties of the related representative spaces, in the sense that their features are independent of the dynamics. From this point of view, the greater generality of the canonical formalism breaks the equivalence between the approaches.
On the other hand, if we only look for the covariance of a particular equation of motion, then Lagrangian and Hamiltonian motions are connected in a well identified way by Legendre mappings.
This dynamical approach is here analyzed by comparing the invariance properties of functions and equations in the two spaces. Technically, what is fundamental is understanding in which way the transformations act on the classical action.
At this point we compare two quotations which involve famous scientists and teaching experts in classical mechanics. The first sentence, where Levi-Civita quotes Birkhoff, is devoted to canonical transformations. The second, due to Goldstein, to canonoid:
"Professor Birkhoff thinks that the premeditated limitation to this particular group of transformations may be regarded as a mere exercise in analytical ingenuity. In my opinion this hard sentence deserves attenuation, and at least temporary amendment."[23]
"... there is added the highly unorthodox, if not downright dangerous, notion of a canonoid transformation - one that is canonical only for certain types of Hamiltonian. (most applications of canonical transformations depend on the property that they be canonical for all Hamiltonians)." [7, p. 430]
Of course both the viewpoints are well-founded and authoritative, but do not take into consideration the possibility to enlarge the concept of invariance of Lagrangian and action.
Acknowledgments
We are particularly indebted to the anonymous referee for all the suggestions necessary to get clear the paper and for drawing our attention to Ref. [21].
Recebido em 18/7/2007; Revisado em 13/11/2007; Aceito em 7/7/2008; Publicado em 18/9/2008
Proof that performing genuine canonoid transformations the Hamiltonian is never a scalar field
Let us write Hamilton equations of motion of the new variables (Q, P), and let us assume that a function K(Q, P) exists such that
Multiplying the first equation by  , the second by
, the second by  and subtracting one has
and subtracting one has
We can find the analogous relation for  , so getting
, so getting
Now, let us assume the scalar invariance of H, so that the right hand sides of Eqs. (50) and (51) are respectively  and
and  . It follows that the fundamental Poisson bracket [Q, P] is equal to 1, and then the transformation is canonical.
. It follows that the fundamental Poisson bracket [Q, P] is equal to 1, and then the transformation is canonical.
We have so proved that the scalar invariance of the Hamiltonian is necessary and sufficient condition for a canonoid map to be canonical.
- [1] R. Talman, Geometric Mechanics (Wiley, Nova Iorque, 2000)
- [2] J.L. McCauley, Classical Mechanics (Cambridge Un. Press, Cambridge, 1997)
- [3] S.F. Singer, Symmetry in Mechanics (Birkhäuser, Boston, 2001)
- [4] G.J. Sussman, J. Wisdom and M.E. Mayer, Structure and Interpretation of Classical Mechanics (MIT Press, Cambridge, 2001).
- [5] J.V. Josè and E.J. Saletan, Classical Dynamics, A Contemporary Approach (Cambridge Un. Press, Cambridge, 1988), p. 201-226.
- [6] E.T. Whittaker, A Treatise on the Analytical Dynamics of Particles and Rigid Bodies (Cambridge Un. Press, Cambridge, 1937), 4th ed.
- [7] H. Goldstein, Classical Mechanics (Addison-Wesley, Reading, 1980), 2nd ed.
- [8] C. Lanczos, The Variational Principles of Mechanics (Toronto Un. Press, Toronto, 1970), 4th ed., p. 161-167.
- [9] G. Marmo, E.J. Saletan, A. Simoni and B. Vitale, Dynamical System: a Differential Geometric Approach to Symmetry and Reduction (Wiley, Chichester, 1985), p. 35-36.
- [10] G. Morandi, C. Ferrario, G. Lo Vecchio, G. Marmo and C. Rubano, Phys. Rep. 188, 147 (1990).
- [11] D.G. Currie and E.J. Saletan, Nuovo Cimento B 9, 143 (1972).
- [12] J.F. Cariñena and M.F. Rañada, J. Math. Phys. 29, 2181 (1988).
- [13] J. Deenen, J. Phys. A: Math.Gen. 14, L273 (1981).
- [14] A. Anderson, Ann. Phys. 232, 292 (1994).
- [15] K.H. Yeon, S.K. Hong, C.I. Um and T.F. George, Int. J. Theor. Phys. 42, 2043 (2003).
- [16] K.H. Yeon, S.K. Hong, C.I. Um and T.F. George, Phys. Lett A 357, 12 (2006).
- [17] B.S. DeWitt, Rev. Mod. Phys. 29, 377 (1957).
- [18] W. Dittrich and M. Reuter, Classical and Quantum Mechanics (Springer-Verlag, Berlin, 2001), 3rd ed., chap. 15.
- [19] G. Esposito, G. Marmo and E.C.G. Sudarshan, From Classical to Quantum Mechanics (Cambridge Un. Press, Cambridge, 2004).
- [20] P. Jordan, Zeit. f. Phys. 37, 383 (1926); P. Jordan, Zeit. f. Phys. 38, 513 (1926).
- [21] A. Jordán and M. Libedinsky, Path Integral Invariance under Point Transformations, hep-th/9703173.
- [22] Y.S. Kim and E.P. Wigner, Am. J. Phys. 58, 439 (1990).
- [23] T. Levi-Civita, Journ. of Math. and Phys. 13, 18 (1934).
Appendix
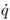 -
-  , but the equivalence between the two formalisms is by now sufficiently clear.
, but the equivalence between the two formalisms is by now sufficiently clear.Publication Dates
-
Publication in this collection
31 Aug 2008 -
Date of issue
Sept 2008
History
-
Received
18 July 2007 -
Reviewed
13 Nov 2007 -
Accepted
07 July 2008












































